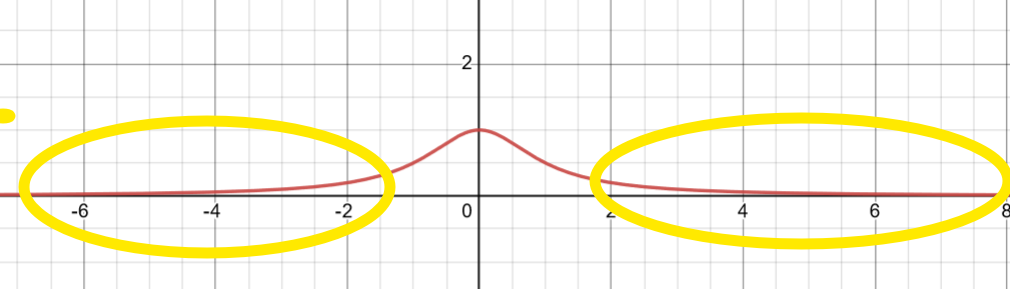
For some quadratic equations, you may never have roots.
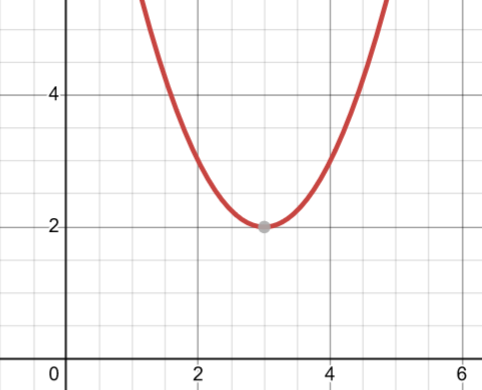reciprocal of this function would be like this:
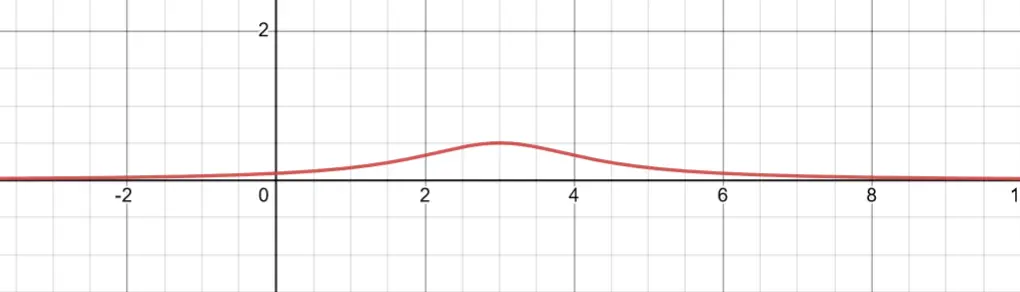As there is no roots, the denominator will never be zero, meaning there are no restrictions for x.
Thus:
The domain is always X ∈ ℝ,
and there are no V.A
Horizontal Shifts
This shift:
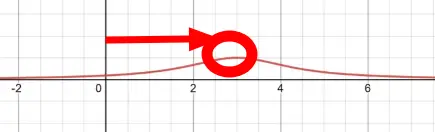Is based on the vertex of the original graph.
It uses the same 1/vertex principle as the other quadratic reciprocal graphs do.
Flipped
Of course, you can also have a negative reciprocal function to result in a flipped graph
Drawing the Quadratic Reciprocal
for a reciprocal g(x) = 1/f(x)
- all you need to find is the min/max point of f(x)
- then, just plot the reciprocal of the min/max point
- Then draw these lines
