Limits

Table Of Contents

${\lim }_{x\to a}f(x)=L$

Its a notation to signify the y value that you get when you x approaches a value. The value of f(x) can be made arbitrarily close to L by choosing x sufficiently close to a but not equal to A

Why Limits?

Limits Can Exist At Discontinuities

A limit would exist at this discontinuity. The left limit will have the same value as the right limit.

Finding Limits

Existence Hints

A limit exists if:

• A valid coordinate exists
• A valid hole coordinate exists. Limits exist at holes They do not exist if:
• Single limits ${\lim }_{x\to a^{-}}\ne {\lim }_{x\to a^{+}}$
  • There is a asymptote at the x value with No cross over point
• Algebra with Limit Properties returns undefined
• They approach infinity
• The value that approach is oscillating between two values (https://www.youtube.com/watch?v=isMZo-OFJOs)
  • ${\lim }_{x\to \infty }\sin x$
  • ${\lim }_{x\to -\infty }\sin x$
  • ${\lim }_{x\to \infty }\cos x$
  • ${\lim }_{x\to -\infty }\cos x$
  • ${\lim }_{x\to -\infty }\tan x$
  • ${\lim }_{x\to -\infty }\tan x$
  • ${\lim }_{x\to \infty }\frac{1}{\sin x}$
  • ${\lim }_{x\to -\infty }\frac{1}{\sin x}$
  • ${\lim }_{x\to \infty }\frac{1}{\cos x}$
  • ${\lim }_{x\to -\infty }\frac{1}{\cos x}$
  • ${\lim }_{x\to \infty }\frac{1}{\tan x}$
  • ${\lim }_{x\to -\infty }\frac{1}{\tan x}$

Algebraic Proof

• Use Algebra with Limit Properties
• You can use direct substitution if there is no hole in the equation

Limit Finding Techniques

• Limit Rationalization
• Limit Substitution
• Limit Division
• Limits with Euler’s Number Squeeze Theorem
• L’Hopital’s Rule

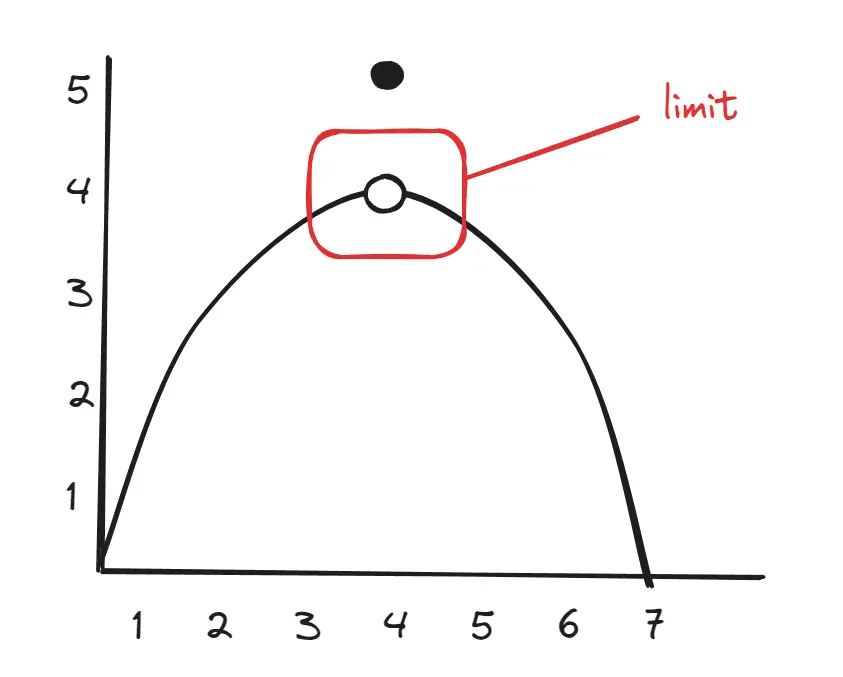
Graphic Proof

• The limit is that point it naturally approaches. Follow the curve, not the jump

Limit Properties

You require the properties of limits to be able to get the limits of any function https://en.wikibooks.org/wiki/Calculus/Proofs_of_Some_Basic_Limit_Rules To use limit rules, you need to prove that each limit used in the limit rule exists. (Unless its for constants and pre-made functions like sin(), cos(), tan(),etc)

Identity Rule

${\lim }_{x\to a}x=a$

Constant Rule

if $a$ and $k$ for any constants then ${\lim }_{x\to a}k=k$

Sums & Difference Rule

The limit of the sum, is the sum of the limits. ${\lim }_{x\to a}[f(x)\pm g(x)]={\lim }_{x\to a}[f(x)]\pm {\lim }_{x\to a}[g(x)]$

Scalar Product Rule

${\lim }_{x\to a}[cf(x)]=c[{\lim }_{x\to a}f(x)]$

Product Rule

${\lim }_{x\to a}[f(x)g(x)]={\lim }_{x\to a}[f(x)]\ast {\lim }_{x\to a}[g(x)]$

Quotient Rule

${\lim }_{x\to a}\left[\frac{f(x)}{g(x)}\right]=\frac{{\lim }_{x\to a}[f(x)]}{{\lim }_{x\to a}[g(x)]}$

Power Rule

Limit of the power is the power of the limit ${\lim }_{x\to a}[f(x{)}^n]={\lim }_{x\to a}[f(x){]}^n$

Composition Rule

If $f$ is continuous then ${\lim }_{x\to a}f(g(x))=f({\lim }_{x\to a}g(x))$

Continuities

Discontinuities

Continuous

For the function to be continuous at a point, 2 conditions:

1. Limit must exist
2. ${\lim }_{x\to a}f(x)=f(a)$

Discontinuous

For the function to be discontinuous at a point, 2 conditions: $li{m}_{x\to a}f(x)\ne f(a)$ OR ${\lim }_{x\to a}f(x)DNE$ OR $L=\pm \infty ORf(x)=\pm \infty$

Additional Concepts

• Limits Formalized Proof
• Limits Does Not Exist