Given a linear map We can express for a given vector as:
Properties
With as the rotation matrix:
Intuition
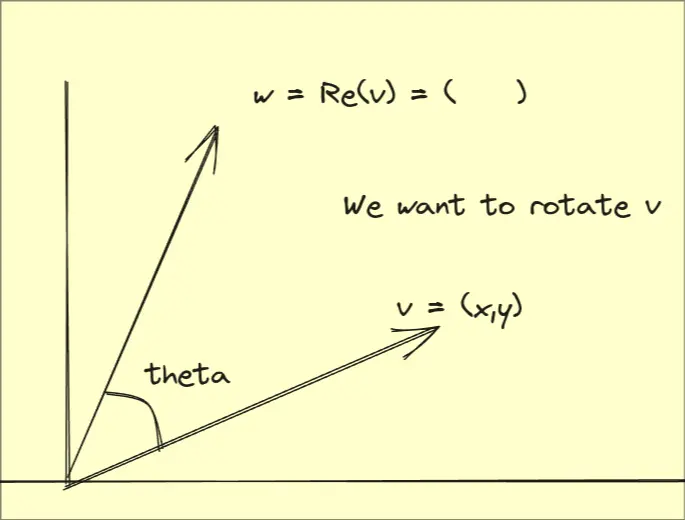
Thus, our final formula is:Drawing 2025-02-07 11.26.28.excalidraw
⚠ Switch to EXCALIDRAW VIEW in the MORE OPTIONS menu of this document. ⚠
Text Elements
v = (x,y)
theta
w = Re(v) = ( )
y = || v || sin(d)
d
y = || v || cos(d)
Supposing v = (x,y) = (||v|| cos(x), ||v|| sin(x)
Then, we have Re(v) = (||v|| cos(d+theta), ||v|| sin(d + theta))
= (||v|| ( cos(d)cos(theta) - sin(d)sin(theta) ), ||v|| (sin(d)cos(theta) + sin(theta)cos(d)) )
by compound angle identity
= ( (||v||cos(d))cos(theta) - (||v||sin(d))sin(theta) , (||v||sin(d))cos(theta) + sin(theta)(||v||cos(d))
= (xcos(theta) - ysin(theta) , ycos(theta) + xsin(theta))
Link to original